Hour 1 & 2 - Introduction to Linear Systems & Gaussian Elimination

Hey everyone! Welcome to our first blog! Firstly, we want to welcome you to MATH 133 and we hope everyone of you will enjoy the learning experience!😃

Now, we all know what linear equations are right? A linear equation is any equation which is in the form a1x1 + a2x2 +…+ anxn + b = 0, where x1, x2,…, xn are variables and a1, a2,…, an and b are real numbers. For example, 2x + 7y = 0 is a linear equation.

Now sometimes, we might be interested to find how linear equations interact with each other. For example, if you have two linear equations: 2x - 3y = 12 and -4x + 6y = -24 and you want to know which values of x and y satisfy both equations, what would you do?

You can plot a graph of both the lines and find the intersecting point to solve it and yes, that’s the geometric way of solving it! But we can also treat it as a system of equations and use matrix form to solve it!

Hour 1

System of equations

We can treat multiple equations together as a system of equations and put them in a matrix form as shown in the picture below:

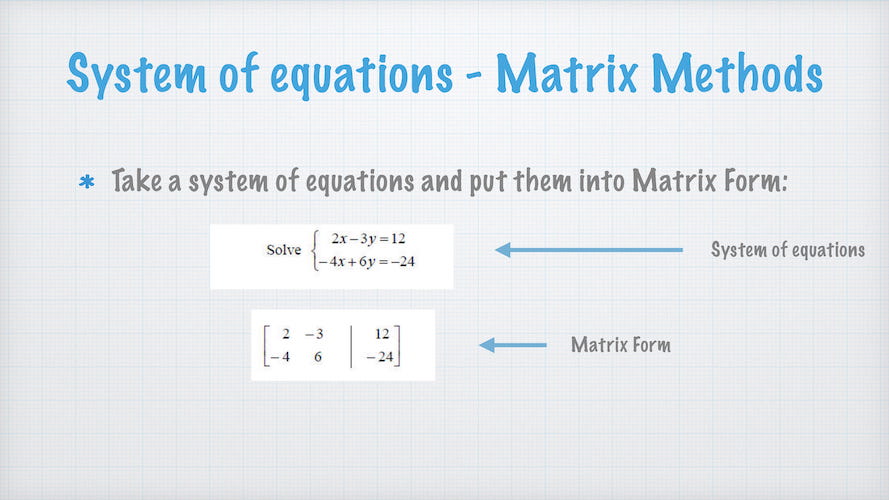
As you can see, the constants are on the right hand side while the coefficients of the variables are used to form a matrix.

Consistent vs Inconsistent Systems

Consistent

A system is consistent if there is atleast one particular solution that satisfies all the equations in the system.

As you can see, in the example above, there is only one solution that satisifies the equations. So, the system is said to be consistent. Moreover, you can also see that the lines intersect, which means there must be a solution!

However, a consistent system can also have many more solutions, infact, infinitely many!

Inconsistent

If a system has no possible solutions, it is said to be inconsistent and you can notice something that the gradients of the lines are the same! It shows that parallel lines will never intersect (except when they are the same line themselves).

Some Helpful Links

Solving Linear Systems of Equation with Matrices:

(1) Tutorial -> https://www.youtube.com/watch?v=AUqeb9Z3y3k

(2) Exercises -> https://www.math-exercises.com/matrices/system-of-equations-solved-by-matrices

Hour 2

Gaussian Elimination

So, we have learnt to write systems of equations in matrix forms. Now, how to solve them? Gaussian Elimination is a process by which we can solve systems of linear equations pretty easily!

Now, to perform Gaussian Elimination, you can do only the following operations:

1. Multiply a row with a non-zero scalar

2. Add / Subtract a row with another

3. Switch the order of the rows

How does a matrix in RREF look like?

A matrix in RREF must have:

a) The first non-zero entry in each row is a leading one, and there are zeroes in the column containing that leading one.

b) The leading one in any row is to the right of the leading one in the row above it.

c) Any rows containing only zeroes appear at the bottom.

Performing the Gaussian Elimination (Examples)

Basically, to perform Gaussian Elimination, we need to make our matrix in it’s reduced row echelon form (rref). The Gaussian Elimination Algorithm is as follows:

a) Find the first column from the left that contains a non-zero entry, “a” and move the row containing this entry “a” to the top.

b) Multiply the new top row by 1/a.

c) Subtract the multiples of that row from other rows to create zeroes.

d) Repeat previous steps on remaining rows.

All this might sound really complicated but the idea is to try to get my matrix to rref in any way by performing the row operations mentioned before.

Some examples here:

So these were some examples of how to solve a system of linear equations using Gaussian Elimination.

Here are some helpful links:

(1) Tutorial

-> https://youtu.be/1rBU0yIyQQ8

-> https://youtu.be/l69YjkuUym0

(2) Exercises

Practice!

-> https://home.cc.umanitoba.ca/~thomas/Courses/RREF_Practice.pdf

-> https://courses.lumenlearning.com/sanjacinto-finitemath1/chapter/2-5-exercises-augmented-matrices/

-> https://www.math-exercises.com/matrices/system-of-equations-solved-by-matrices

So far, we have covered linear systems and how to solve them using Gaussian Elimination and I would like to thank my wonderful co-TA Sophia for the amazing slides! Stay tuned for the next blog! 😃